Main Page: Difference between revisions
No edit summary |
No edit summary |
||
| Line 1: | Line 1: | ||
In [[multivariable calculus]], the '''implicit function theorem''' is a tool which allows [[relation (mathematics)#Formal definition|relations]] to be converted to [[function (mathematics)|function]]s. It does this by representing the relation as the [[graph of a function]]. There may not be a single function whose graph is the entire relation, but there may be such a function on a restriction of the [[domain (mathematics)|domain]] of the relation. The implicit function theorem gives a sufficient condition to ensure that there is such a function. | |||
= | The theorem states that if the equation <math>R(x, y) = 0</math> (an [[implicit function]]) satisfies some mild conditions on its [[partial derivative]]s, then one can in principle solve this equation for <math>y</math>, at least over some small [[interval (mathematics)|interval]]. Geometrically, the [[Locus (mathematics)|locus]] defined by <math>R(x, y) = 0</math> will overlap [[local property|locally]] with the graph of a function <math>y = f(x)</math> (an explicit function, see article on [[implicit function]]s). | ||
[[ | |||
< | |||
</ | |||
The | == First example == | ||
[[Image:Implicit circle.svg|thumb|right|200px|The unit circle can be specified as the level curve <math>f(x,y)=1</math> of the function <math>f(x,y)=x^2 + y^2</math>. Around point A, ''y'' can be expressed as a function <math>y(x)</math>, specifically <math>g_1(x)=\sqrt{1-x^2}</math>. No such function exists around point B.]] | |||
If we define the function <math>f(x,y)=x^2 + y^2</math>, then the equation <math>f(x,y)=1</math> cuts out the [[unit circle]] as the [[level set]] <math>\{ (x,y) | f(x,y) = 1 \}</math>. There is no way to represent the unit circle as the graph of a function of one variable <math>y = g(x)</math> because for each choice of <math>x \in (-1,1),</math> there are two choices of <math>y</math>, namely <math>\pm\sqrt{1-x^2}</math>. | |||
== | However, it is possible to represent part of the circle as the graph of a function of one variable. If we let <math>g_1(x) = \sqrt{1-x^2}</math> for <math>-1 < x < 1</math>, then the graph of <math>y = g_1(x)</math> provides the upper half of the circle. Similarly, if <math>g_2(x) = -\sqrt{1-x^2}</math>, then the graph of <math>y = g_2(x)</math> gives the lower half of the circle. | ||
The purpose of the implicit function theorem is to tell us the existence of functions like <math>g_1(x)</math> and <math>g_2(x)</math>, even in situations where we cannot write down explicit formulas. It guarantees that <math>g_1(x)</math> and <math>g_2(x)</math> are differentiable, and it even works in situations where we do not have a formula for <math>f(x,y)</math>. | |||
< | |||
</ | |||
:< | == Statement of the theorem == | ||
Let ''f'' : '''R'''<sup>''n+m''</sup> → '''R'''<sup>''m''</sup> be a [[continuously differentiable]] function. We think of '''R'''<sup>''n+m''</sup> as the [[Cartesian product]] '''R'''<sup>''n''</sup> × '''R'''<sup>''m''</sup>, and we write a point of this product as ('''x''','''y''') = (''x<sub>1</sub>'', ..., ''x<sub>n</sub>'', ''y<sub>1</sub>'', ..., ''y<sub>m</sub>''). Starting from the given function ''f'', our goal is to construct a function ''g'' : '''R'''<sup>''n''</sup> → '''R'''<sup>''m''</sup> whose graph ('''x''', g('''x''')) is precisely the set of all ('''x''', '''y''') such that ''f''('''x''', '''y''') = '''0'''. | |||
:< | |||
As noted above, this may not always be possible. We will therefore fix a point ('''a''','''b''') = (''a<sub>1</sub>'', ..., ''a<sub>n</sub>'', ''b<sub>1</sub>'', ..., ''b<sub>m</sub>'') which satisfies ''f''('''a''', '''b''') = 0, and we will ask for a ''g'' that works near the point ('''a''', '''b'''). In other words, we want an [[open set]] ''U'' of '''R'''<sup>''n''</sup>, an open set ''V'' of '''R'''<sup>''m''</sup>, and a function ''g'' : ''U'' → ''V'' such that the graph of ''g'' satisfies the relation ''f'' = 0 on ''U'' × ''V''. In symbols, | |||
: | :<math>\{ (\mathbf{x}, g(\mathbf{x})) \mid \mathbf x \in U \} = \{ (\mathbf{x}, \mathbf{y})\in U \times V \mid f(\mathbf{x}, \mathbf{y}) = 0 \}.</math> | ||
This | To state the implicit function theorem, we need the [[Jacobian matrix and determinant|Jacobian]], also called the ''differential'' or ''total derivative'', of <math>f</math>. This is the matrix of [[partial derivative]]s of <math>f</math>. Abbreviating (''a''<sub>1</sub>, ..., ''a''<sub>n</sub>, ''b''<sub>1</sub>, ..., ''b''<sub>m</sub>) to ('''a''', '''b'''), the Jacobian matrix is | ||
:<math>\begin{matrix} | |||
(Df)(\mathbf{a},\mathbf{b}) & = & | |||
\left[\begin{matrix} | |||
\frac{\partial f_1}{\partial x_1}(\mathbf{a},\mathbf{b}) & | |||
\cdots & \frac{\partial f_1}{\partial x_n}(\mathbf{a},\mathbf{b})\\ | |||
\vdots & \ddots & \vdots\\ | |||
\frac{\partial f_m}{\partial x_1}(\mathbf{a},\mathbf{b}) & \cdots & \frac{\partial f_m}{\partial x_n}(\mathbf{a},\mathbf{b}) | |||
\end{matrix}\right|\left. | |||
\begin{matrix} | |||
\frac{\partial f_1}{\partial y_1}(\mathbf{a},\mathbf{b}) & \cdots & \frac{\partial f_1}{\partial y_m}(\mathbf{a},\mathbf{b})\\ | |||
\vdots & \ddots & \vdots\\ | |||
\frac{\partial f_m}{\partial y_1}(\mathbf{a},\mathbf{b}) & \cdots & \frac{\partial f_m}{\partial y_m}(\mathbf{a},\mathbf{b})\\ | |||
\end{matrix}\right]\\ | |||
& = & \begin{bmatrix} X & | & Y \end{bmatrix}\\ | |||
\end{matrix}</math> | |||
where <math>X</math> is the matrix of partial derivatives in the <math>x</math>'s and <math>Y</math> is the matrix of partial derivatives in the <math>y</math>'s. The implicit function theorem says that if <math>Y</math> is an invertible matrix, then there are <math>U</math>, <math>V</math>, and <math>g</math> as desired. Writing all the hypotheses together gives the following statement. | |||
''' | :Let ''f'' : '''R'''<sup>n+m</sup> → '''R'''<sup>m</sup> be a [[continuously differentiable]] function, and let '''R'''<sup>n+m</sup> have coordinates ('''x''', '''y'''). Fix a point (''a''<sub>1</sub>,...,''a''<sub>n</sub>,''b''<sub>1</sub>,...,''b''<sub>m</sub>) = ('''a'',''b''') with ''f''('''a''','''b''')='''c''', where '''c'''∈ '''R'''<sup>m</sup>. If the matrix [(∂''f''<sub>i</sub>/∂''y''<sub>j</sub>)('''a''','''b''')] is [[invertible]], then there exists an open set ''U'' containing '''a''', an open set ''V'' containing '''b''', and a unique continuously differentiable function ''g'':''U'' → ''V'' such that | ||
::<math>\{ (\mathbf{x}, g(\mathbf{x}))|\mathbf x \in U \} = \{ (\mathbf{x}, \mathbf{y}) \in U \times V| f(\mathbf{x}, \mathbf{y}) = \mathbf{c} \}.</math> | |||
===Regularity=== | |||
= | It can be proven that whenever we have the additional hypothesis that ''f'' is continuously differentiable up to ''k'' times inside ''U×V'', then the same holds true for the explicit function ''g'' inside ''U'' and | ||
{{ | :<math>\frac{\partial g}{\partial x_j}(x)=-\left( \frac{\partial f}{\partial y}(x,g(x)) \right)^{-1} \frac{\partial f}{\partial x_j}(x,g(x)) </math>. | ||
== | Similarly, if ''f'' is [[analytic function|analytic]] inside ''U×V'', then the same holds true for the explicit function ''g'' inside ''U''.<ref> K. Fritzsche, H. Grauert (2002), [http://books.google.de/books?id=jSeRz36zXIMC&lpg=PP1&dq=fritzsche%20grauert&hl=de&pg=PA34#v=onepage&q&f=false "From Holomorphic Functions to Complex Manifolds"], Springer-Verlag, page 34. </ref> | ||
{{ | This generalization is called the '''analytic implicit function theorem'''. | ||
== The circle example == | |||
Let us go back to the example of the [[unit circle]]. In this case <math> n=m=1 </math> and <math>f(x,y) = x^2 + y^2 - 1</math>. The matrix of partial derivatives is just a 1×2 matrix, given by | |||
:<math>\begin{matrix} | |||
(Df)(a,b) & = & \begin{bmatrix} | |||
\frac{\partial f}{\partial x}(a,b) & \frac{\partial f}{\partial y}(a,b)\\ | |||
\end{bmatrix}\\ | |||
& = & \begin{bmatrix} 2a & 2b \end{bmatrix}.\\ | |||
\end{matrix}</math> | |||
Thus, here, <math>Y</math> is just a number; the linear map defined by it is invertible [[iff]] <math>b \neq 0 </math>. By the implicit function theorem we see that we can write the circle in the form <math> y=g(x) </math> for all points where <math> y\neq 0 </math>. For <math> (-1,0) </math> and <math> (1,0) </math> we run into trouble, as noted before. | |||
== Application: change of coordinates == | |||
Suppose we have an m-dimensional space, parametrised by a set of coordinates <math> (x_1,\ldots,x_m) </math>. We can introduce a new coordinate system <math> (x'_1,\ldots,x'_m) </math> by supplying m functions <math> h_1\ldots h_m </math>. These functions allow to calculate the new coordinates <math> (x'_1,\ldots,x'_m) </math> of a point, given the point's old coordinates <math> (x_1,\ldots,x_m) </math> using <math> x'_1=h_1(x_1,\ldots,x_m), \ldots, x'_m=h_m(x_1,\ldots,x_m) </math>. One might want to verify if the opposite is possible: given coordinates <math> (x'_1,\ldots,x'_m) </math>, can we 'go back' and calculate the same point's original coordinates <math> (x_1,\ldots,x_m) </math>? The implicit function theorem will provide an answer to this question. The (new and old) coordinates <math>(x'_1,\ldots,x'_m, x_1,\ldots,x_m)</math> are related by <math> f=0 </math>, with | |||
:<math> | |||
f(x'_1,\ldots,x'_m,x_1,\ldots x_m)=(h_1(x_1,\ldots x_m)-x'_1,\ldots , h_m(x_1,\ldots, x_m)-x'_m). | |||
</math> | |||
Now the Jacobian matrix of ''f'' at a certain point <math>(a,b)</math> [ where <math>a=(x'_1,\ldots,x'_m), b=(x_1,\ldots,x_m)</math> ] is given by | |||
:<math>\begin{matrix} | |||
(Df)(a,b) & = & \begin{bmatrix} | |||
-1 & \cdots & 0 & \frac{\partial h_1}{\partial x_1}(b) & \cdots & \frac{\partial h_1}{\partial x_m}(b)\\ | |||
\vdots & \ddots & \vdots & \vdots & \ddots & \vdots\\ | |||
0 & \cdots & -1 & \frac{\partial h_m}{\partial x_1}(b) & \cdots & \frac{\partial h_m}{\partial x_m}(b)\\ | |||
\end{bmatrix}\\ | |||
& = & \begin{bmatrix} -1_m & | & J \end{bmatrix}.\\ | |||
\end{matrix}</math> | |||
where <math>1_m</math> denotes the <math>m\times m</math> [[identity matrix]], and J is the <math>m\times m</math> matrix of partial derivatives, evaluated at <math>(a,b)</math>. (In the above, these blocks were denoted by X and Y. As it happens, in this particular application of the theorem, neither matrix depends on <math>a</math>.) The implicit function theorem now states that we can locally express <math> (x_1,\ldots,x_m) </math> as a function of <math> (x'_1,\ldots,x'_m) </math> if J is invertible. Demanding J is invertible is equivalent to <math> \det J \neq 0 </math>, thus we see that we can go back from the primed to the unprimed coordinates if the determinant of the Jacobian J is non-zero. This statement is also known as the [[inverse function theorem]]. | |||
=== Example: polar coordinates === | |||
As a simple application of the above, consider the plane, parametrised by [[polar coordinates]] <math> (R,\theta) </math>. We can go to a new coordinate system ([[cartesian coordinates]]) by defining functions <math>x(R,\theta)=R \cos \theta </math> and | |||
<math>y(R,\theta)=R \sin \theta </math>. This makes it possible given any point <math> (R,\theta) </math> to find corresponding cartesian coordinates <math> (x,y) </math>. When can we go back and convert cartesian into polar coordinates? By the previous example, we need <math> \det J \neq 0 </math>, with | |||
:<math> | |||
J =\begin{bmatrix} | |||
\frac{\partial x(R,\theta)}{\partial R} & \frac{\partial x(R,\theta)}{\partial \theta} \\ | |||
\frac{\partial y(R,\theta)}{\partial R} & \frac{\partial y(R,\theta)}{\partial \theta} \\ | |||
\end{bmatrix}= | |||
\begin{bmatrix} | |||
\cos \theta & -R \sin \theta \\ | |||
\sin \theta & R \cos \theta | |||
\end{bmatrix}. | |||
</math> | |||
Since <math> \det J = R </math>, the conversion back to polar coordinates is only possible if <math> R\neq 0 </math>. This is a consequence of the fact that at the origin, polar coordinates don't exist: the value of <math> \theta </math> | |||
is not well-defined. | |||
== Generalizations == | |||
=== Banach space version === | |||
Based on the [[inverse function theorem]] in [[Banach space]]s, it is possible to extend the implicit function theorem to Banach space valued mappings. | |||
Let <math>X</math>, <math>Y</math>, <math>Z</math> be [[Banach space]]s. Let the mapping <math>f:X\times Y\to Z</math> be continuously [[Frechet differentiable|Fréchet differentiable]]. If <math>(x_0,y_0)\in X\times Y</math>, <math>f(x_0,y_0)=0</math>, and <math>y\mapsto Df(x_0,y_0)(0,y)</math> is a Banach space isomorphism from <math>Y</math> onto <math>Z</math>, then there exist neighbourhoods <math>U</math> of <math>x_0</math> and <math>V</math> of <math>y_0</math> and a Frechet differentiable function <math>g:U\to V</math> such that <math>f(x,g(x))=0</math> and <math>f(x,y)=0</math> if and only if <math>y=g(x)</math>, for all <math>(x,y)\in U\times V</math>. | |||
=== Implicit functions from non-differentiable functions === | |||
Various forms of the implicit function theorem exist for the case when the function <math>f</math> is not differentiable. It is standard that it holds in one dimension.<ref>L. D. Kudryavtsev, "Implicit function" in Encyclopedia of Mathematics,M. Hazewinkel, Ed. Dordrecht, The Netherlands: Kluwer, 1990.</ref> The following more general form was proven by Kumagai<ref>S. Kumagai, "An implicit function theorem: Comment," ''Journal of Optimization Theory and Applications'', 31(2):285-288, June 1980.</ref> based on an observation by Jittorntrum.<ref>K. Jittorntrum, "An Implicit Function Theorem", ''Journal of Optimization Theory and Applications'', 25(4), 1978.</ref> | |||
Consider a continuous function <math>f : R^n \times R^m \rightarrow R^n</math> such that <math>f(x_0, y_0) = 0</math>. '''If''' there exist open neighbourhoods <math>A \subset R^n</math> and <math>B \subset R^m</math> of <math>x_0</math> and <math>y_0</math>, respectively, such that, for all <math>y \in B</math>, <math>f(\cdot, y) : A \rightarrow R^n</math> is locally one-to-one '''then''' there exist open neighbourhoods <math>A_0 \subset R^n</math> and <math>B_0 \subset R^m</math> of <math>x_0</math> and <math>y_0</math>, | |||
such that, for all <math>y \in B_0</math>, the equation | |||
:<math>f(x, y) = 0</math> | |||
has a unique solution | |||
:<math>x = g(y) \in A_0</math>, | |||
where <math>g</math> is a continuous function from <math>B_0</math> into <math>A_0</math>. | |||
== | == See also == | ||
*[[Constant rank theorem]]: Both the implicit function theorem and the [[Inverse function theorem]] can be seen as special cases of the constant rank theorem. | |||
==Notes== | |||
{{refs}} | |||
{{ | == References == | ||
{{ | *{{springer | ||
| title=Implicit function | |||
| id= i/i050310 | |||
| last= Kudryavtsev | |||
| first= L.D. | |||
| author-link=Lev Dmitrievich Kudryavtsev | |||
}} | |||
*{{springer | |||
| title=Implicit function (in algebraic geometry) | |||
| id= i/i050320 | |||
| last= Danilov | |||
| first= V.I. | |||
| author-link= | |||
}} | |||
[[Category: | {{DEFAULTSORT:Implicit Function Theorem}} | ||
[[Category:Theorems in calculus]] | |||
[[Category:Theorems in real analysis]] | |||
[[ca:Teorema de la funció implícita]] | |||
[[de:Satz von der impliziten Funktion]] | |||
[[es:Teorema de la función implícita]] | |||
[[fr:Théorème des fonctions implicites]] | |||
[[ca: | [[gl:Teorema da función implícita]] | ||
[[ko:음함수 정리]] | |||
[[de: | [[it:Teorema delle funzioni implicite]] | ||
[[he:משפט הפונקציות הסתומות]] | |||
[[kk:Айқындалмаған функция]] | |||
[[es: | [[hu:Implicitfüggvény-tétel]] | ||
[[nl:Impliciete functiestelling]] | |||
[[fr: | [[ru:Теорема о неявной функции]] | ||
[[scn:Tiurema dâ funzioni mplicita]] | |||
[[sv:Implicita funktionssatsen]] | |||
[[gl: | [[uk:Теорема про неявну функцію]] | ||
[[ | [[zh:隐函数定理]] | ||
[[it: | |||
[[he: | |||
[[ | |||
[[ | |||
[[ | |||
[[ru: | |||
[[ | |||
[[sv: | |||
[[uk: | |||
[[zh: | |||
Revision as of 10:05, 12 August 2014
In multivariable calculus, the implicit function theorem is a tool which allows relations to be converted to functions. It does this by representing the relation as the graph of a function. There may not be a single function whose graph is the entire relation, but there may be such a function on a restriction of the domain of the relation. The implicit function theorem gives a sufficient condition to ensure that there is such a function.
The theorem states that if the equation (an implicit function) satisfies some mild conditions on its partial derivatives, then one can in principle solve this equation for , at least over some small interval. Geometrically, the locus defined by will overlap locally with the graph of a function (an explicit function, see article on implicit functions).
First example
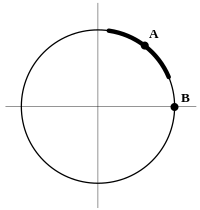
If we define the function , then the equation cuts out the unit circle as the level set . There is no way to represent the unit circle as the graph of a function of one variable because for each choice of there are two choices of , namely .
However, it is possible to represent part of the circle as the graph of a function of one variable. If we let for , then the graph of provides the upper half of the circle. Similarly, if , then the graph of gives the lower half of the circle.
The purpose of the implicit function theorem is to tell us the existence of functions like and , even in situations where we cannot write down explicit formulas. It guarantees that and are differentiable, and it even works in situations where we do not have a formula for .
Statement of the theorem
Let f : Rn+m → Rm be a continuously differentiable function. We think of Rn+m as the Cartesian product Rn × Rm, and we write a point of this product as (x,y) = (x1, ..., xn, y1, ..., ym). Starting from the given function f, our goal is to construct a function g : Rn → Rm whose graph (x, g(x)) is precisely the set of all (x, y) such that f(x, y) = 0.
As noted above, this may not always be possible. We will therefore fix a point (a,b) = (a1, ..., an, b1, ..., bm) which satisfies f(a, b) = 0, and we will ask for a g that works near the point (a, b). In other words, we want an open set U of Rn, an open set V of Rm, and a function g : U → V such that the graph of g satisfies the relation f = 0 on U × V. In symbols,
To state the implicit function theorem, we need the Jacobian, also called the differential or total derivative, of . This is the matrix of partial derivatives of . Abbreviating (a1, ..., an, b1, ..., bm) to (a, b), the Jacobian matrix is
where is the matrix of partial derivatives in the 's and is the matrix of partial derivatives in the 's. The implicit function theorem says that if is an invertible matrix, then there are , , and as desired. Writing all the hypotheses together gives the following statement.
- Let f : Rn+m → Rm be a continuously differentiable function, and let Rn+m have coordinates (x, y). Fix a point (a1,...,an,b1,...,bm) = (a,b) with f(a,b)=c, where c∈ Rm. If the matrix [(∂fi/∂yj)(a,b)] is invertible, then there exists an open set U containing a, an open set V containing b, and a unique continuously differentiable function g:U → V such that
Regularity
It can be proven that whenever we have the additional hypothesis that f is continuously differentiable up to k times inside U×V, then the same holds true for the explicit function g inside U and
Similarly, if f is analytic inside U×V, then the same holds true for the explicit function g inside U.[1] This generalization is called the analytic implicit function theorem.
The circle example
Let us go back to the example of the unit circle. In this case and . The matrix of partial derivatives is just a 1×2 matrix, given by
Thus, here, is just a number; the linear map defined by it is invertible iff . By the implicit function theorem we see that we can write the circle in the form for all points where . For and we run into trouble, as noted before.
Application: change of coordinates
Suppose we have an m-dimensional space, parametrised by a set of coordinates . We can introduce a new coordinate system by supplying m functions . These functions allow to calculate the new coordinates of a point, given the point's old coordinates using . One might want to verify if the opposite is possible: given coordinates , can we 'go back' and calculate the same point's original coordinates ? The implicit function theorem will provide an answer to this question. The (new and old) coordinates are related by , with
Now the Jacobian matrix of f at a certain point [ where ] is given by
where denotes the identity matrix, and J is the matrix of partial derivatives, evaluated at . (In the above, these blocks were denoted by X and Y. As it happens, in this particular application of the theorem, neither matrix depends on .) The implicit function theorem now states that we can locally express as a function of if J is invertible. Demanding J is invertible is equivalent to , thus we see that we can go back from the primed to the unprimed coordinates if the determinant of the Jacobian J is non-zero. This statement is also known as the inverse function theorem.
Example: polar coordinates
As a simple application of the above, consider the plane, parametrised by polar coordinates . We can go to a new coordinate system (cartesian coordinates) by defining functions and . This makes it possible given any point to find corresponding cartesian coordinates . When can we go back and convert cartesian into polar coordinates? By the previous example, we need , with
Since , the conversion back to polar coordinates is only possible if . This is a consequence of the fact that at the origin, polar coordinates don't exist: the value of is not well-defined.
Generalizations
Banach space version
Based on the inverse function theorem in Banach spaces, it is possible to extend the implicit function theorem to Banach space valued mappings.
Let , , be Banach spaces. Let the mapping be continuously Fréchet differentiable. If , , and is a Banach space isomorphism from onto , then there exist neighbourhoods of and of and a Frechet differentiable function such that and if and only if , for all .
Implicit functions from non-differentiable functions
Various forms of the implicit function theorem exist for the case when the function is not differentiable. It is standard that it holds in one dimension.[2] The following more general form was proven by Kumagai[3] based on an observation by Jittorntrum.[4]
Consider a continuous function such that . If there exist open neighbourhoods and of and , respectively, such that, for all , is locally one-to-one then there exist open neighbourhoods and of and , such that, for all , the equation
has a unique solution
where is a continuous function from into .
See also
- Constant rank theorem: Both the implicit function theorem and the Inverse function theorem can be seen as special cases of the constant rank theorem.
Notes
References
- Other Sports Official Kull from Drumheller, has hobbies such as telescopes, property developers in singapore and crocheting. Identified some interesting places having spent 4 months at Saloum Delta.
my web-site http://himerka.com/ - Other Sports Official Kull from Drumheller, has hobbies such as telescopes, property developers in singapore and crocheting. Identified some interesting places having spent 4 months at Saloum Delta.
my web-site http://himerka.com/
ca:Teorema de la funció implícita de:Satz von der impliziten Funktion es:Teorema de la función implícita fr:Théorème des fonctions implicites gl:Teorema da función implícita ko:음함수 정리 it:Teorema delle funzioni implicite he:משפט הפונקציות הסתומות kk:Айқындалмаған функция hu:Implicitfüggvény-tétel nl:Impliciete functiestelling ru:Теорема о неявной функции scn:Tiurema dâ funzioni mplicita sv:Implicita funktionssatsen uk:Теорема про неявну функцію zh:隐函数定理
- ↑ K. Fritzsche, H. Grauert (2002), "From Holomorphic Functions to Complex Manifolds", Springer-Verlag, page 34.
- ↑ L. D. Kudryavtsev, "Implicit function" in Encyclopedia of Mathematics,M. Hazewinkel, Ed. Dordrecht, The Netherlands: Kluwer, 1990.
- ↑ S. Kumagai, "An implicit function theorem: Comment," Journal of Optimization Theory and Applications, 31(2):285-288, June 1980.
- ↑ K. Jittorntrum, "An Implicit Function Theorem", Journal of Optimization Theory and Applications, 25(4), 1978.




















![{\displaystyle {\begin{matrix}(Df)(\mathbf {a} ,\mathbf {b} )&=&\left[{\begin{matrix}{\frac {\partial f_{1}}{\partial x_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\frac {\partial f_{1}}{\partial x_{n}}}(\mathbf {a} ,\mathbf {b} )\\\vdots &\ddots &\vdots \\{\frac {\partial f_{m}}{\partial x_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\frac {\partial f_{m}}{\partial x_{n}}}(\mathbf {a} ,\mathbf {b} )\end{matrix}}\right|\left.{\begin{matrix}{\frac {\partial f_{1}}{\partial y_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\frac {\partial f_{1}}{\partial y_{m}}}(\mathbf {a} ,\mathbf {b} )\\\vdots &\ddots &\vdots \\{\frac {\partial f_{m}}{\partial y_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\frac {\partial f_{m}}{\partial y_{m}}}(\mathbf {a} ,\mathbf {b} )\\\end{matrix}}\right]\\&=&{\begin{bmatrix}X&|&Y\end{bmatrix}}\\\end{matrix}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d354fbe7862bb7385edcd697ded1401c7b7fe981)


























































